Non-attacking queens in an n by n by n cube

It’s possible to place n queens on an n × n chess board so that no queen is attacking any other queen, provided n > 3. A queen is able to move any number of squares in any horizontal, vertical, or diagonal direction. Another way to put it is that the queen can move in any of 8 = 3² − 1 directions, in the direction of any cell in a 3 × 3 grid centered on the queen.

What if we extend chess to three dimensions? Now we have an n × n × n cube. Now a queen is able to move in 26 = 3³ − 1 directions, in the direction of any cell in a 3 × 3 × 3 cube centered on the queen.

Klarner [1] proved that it is possible to place n² queens in an n × n × n cube so that no queen is attacking any other, provided gcd(n, 210) = 1, i.e. provided the largest prime factor of n is larger than 7. The condition gcd(n, 210) = 1 is sufficient, and it is conjectured to be necessary as well [2].

Klarner constructed a solution as follows: Place the queens on

(i, j, (3i + 5j) mod n)

for i and j running from 1 to n.

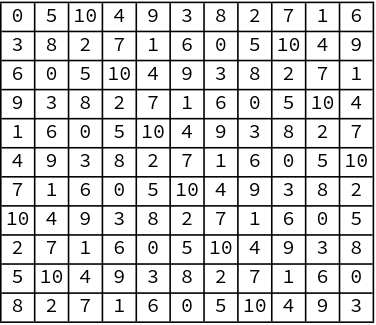
One way to visualize the queens in 3D is to draw a 2D grid where each cell contains the vertical coordinate of the corresponding queen. This grid will be a Latin square, and so the 3D queen placement problem is also known as the Latin queen problem.

Here’s a visualization of Klarner’s example.

Note that if you only pay attention to a particular number, you have a solution to the 11 queens problem in a square. That’s because every slice of a 3D solution is a solution to the 2D problem.

I played around with plotting the points in three dimensions. Here’s one view.

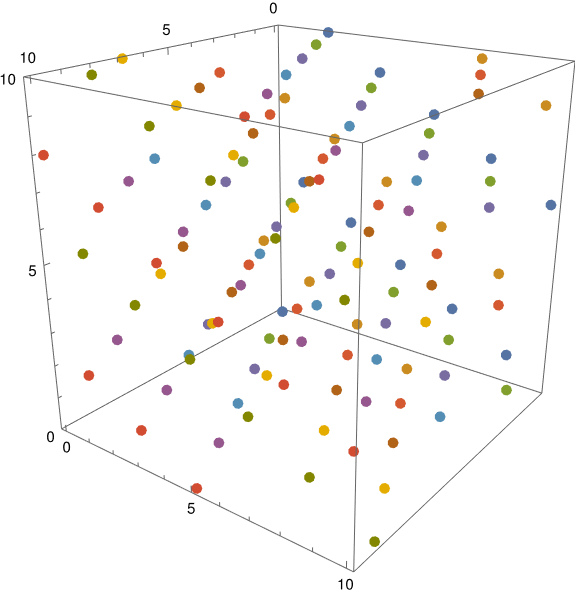
A slight rotation of the cube gives a substantially different perspective. This would be better as an animation, available here.

Mathematica code

Here’s the code that made the images above.

Flat grid:

    Grid[
        Table[
            Mod[3 i + 5 j, 11], 
            {i, 0, 10}, 
            {j, 0, 10}
        ], 
        Frame -> All
    ]

Static 3D view:

     ListPointPlot3D[
         Table[
             {i, j, Mod[3 i + 5 j, 11]}, 
             {i, 0, 10}, 
             {j, 0, 10}
          ], 
          BoxRatios -> {1, 1, 1}, 
          PlotStyle -> {PointSize[0.02]}
      ]

Animation:

    Animate[
        ListPointPlot3D[
            Table[
                {i, j, Mod[3 i + 5 j, 11]}, 
                {i, 0, 10}, 
                {j, 0, 10}
            ], 
        BoxRatios -> {1, 1, 1}, 
        PlotStyle -> {PointSize[0.02]}, 
        ViewPoint -> {2 Cos[t], 2 Sin[t], 1}
        ], 
        {t, 0, Pi, 0.05}, 
        AnimationRunning -> True, 
        AnimationRate -> 4
    ]

    Export["klerner_animation.gif", %]

Related posts

[1] D.A. Klarner, Queen squares, J. Recreational Math. 12 (3) (1979/1980) 177–178.

[2] Jordan Bell and Brett Stevens. A survey of known results and research areas for n-queens. Discrete Mathematics 309 (2009) 1–31